instances of Fox's theorem
Fox’s theorem comes in multiple versions: two of them describe the construction of the cofree and the free cartesian category over a monoidal category. The more compact formulation says that any symmetric monoidal category is a cartesian category exactly if each object is a cocommutative comonoid and each morphism is a comonoid homomorphism, in a natural and uniform way.
The adjunctions of Fox’s theorem are hom-tensor adjunctions between the tensor of monoidal categories and the exponential of symmetric monoidal categories. These explain the requirements for naturality and uniformity in Fox’s theorem.
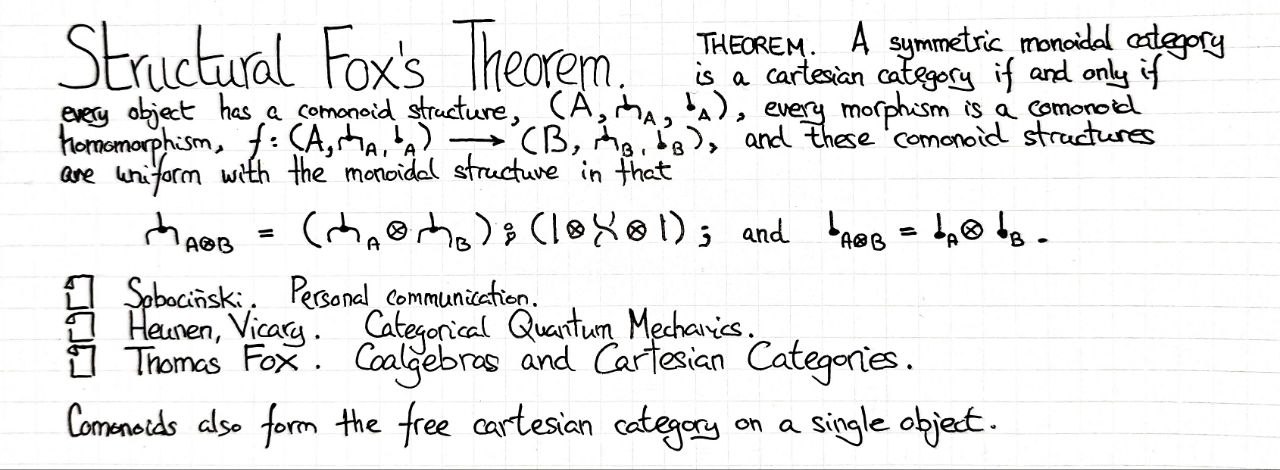
References
Instances of Fox’s theorem.
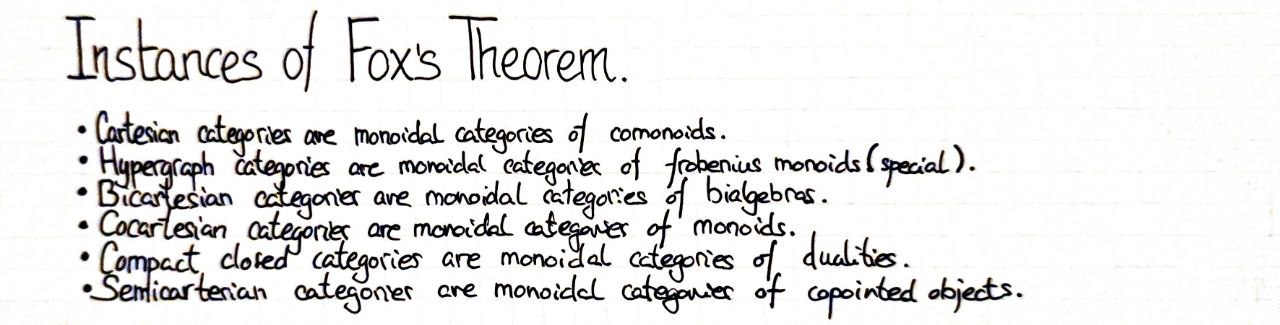
See: Fox’s theorem.
