pseudomonoids of multivariable adjunctions are closed categories
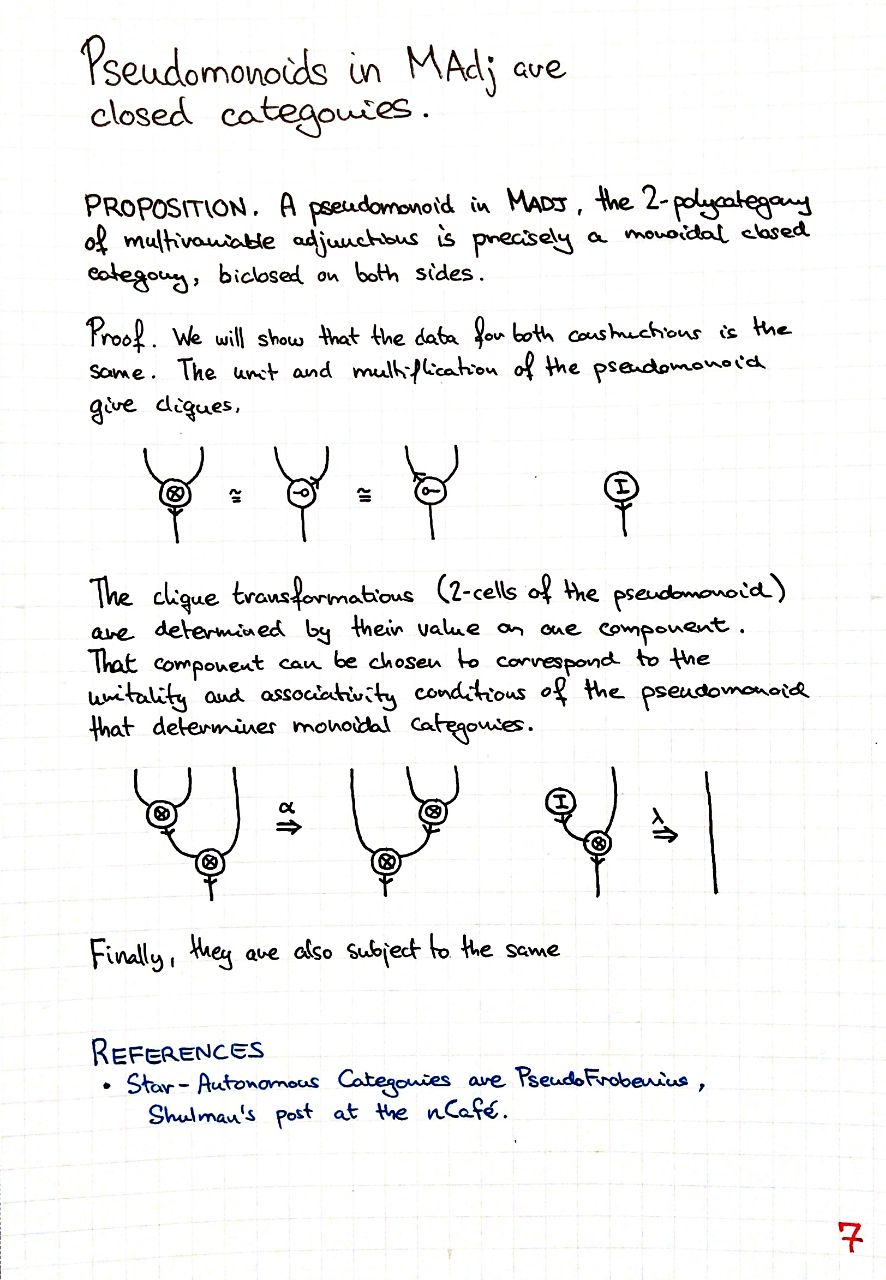
Proposition. A pseudomonoid in the 2-polycategory of multivariable adjunctions is precisely a monoidal biclosed category.
Proof. We will show that the data for both constructions is the same. The unit and multiplication of the pseudomonoid give cliques representing the tensor with a left and right adjoint.
The clique transformations (2-cells of the pseudomonoid) are determined by their value on one component. That component can be chosen to correspond to the unitality and associativity conditions of the pseudomonoid that determines monoidal categories.
Finally, they are also subject to the same coherence conditions.
Tags: pseudomonoid, closed monoidal category, adjunction - multivariable adjunction