Polycategorical splice
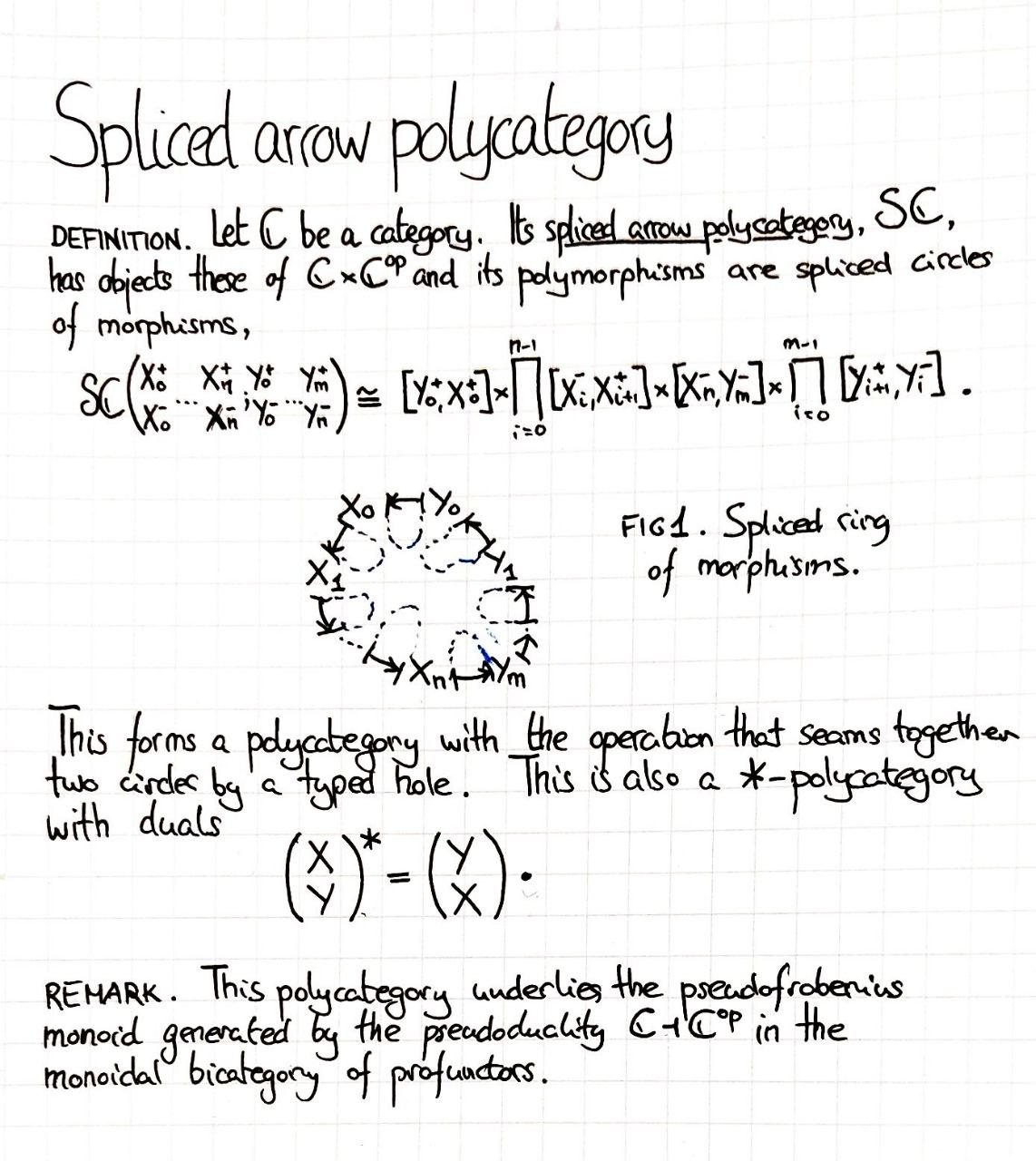The spliced arrow
polycategory generalizes the
promonoidal category of spliced arrows. It is right adjoint to
polycategorical contour.
# Definition
Definition. Let ℂ be a category. Its spliced arrow $\star$-polycategory, $𝓢ₚℂ$, has objects these of $ℂ×ℂ^{op}$ and its polymorphisms are given by $$ 𝓢ₚℂ \left( \binom{X₀^{+}}{X₀^{-}}, \dots, \binom{Xₙ^{+}}{Xₙ^{-}}; \binom{Y₀^{+}}{Y₀^{-}}, \dots, \binom{Yₘ^{+}}{Yₘ^{-}}; \right) = [Y₀^{+},X₀^{+}] × [Xₙ^{-},Yₘ^{-}] × \prod_{i=0}^{n-1} [Xᵢ^{-},X_{i+1}^{+}] × \prod_{i=0}^{n-1} [Y_{i+1}^{+},Y_{i}^{-}]. $$ An intuition is that polymorphisms are spliced circles of morphisms. Composition glues together two of these circles along a typed hole. Duals are given by interchanging the top and bottom objects.
Remark. This polycategory underlies the Frobenius pseudomonoid generated by the pseudoduality $ℂ \dashv ℂ^{op}$ in the monoidal bicategory of profunctors.